pp. 27-36
Dr. Piynsha Somvanshi
S. Jain Subhodh P. G. (Autonomous) College, Rambagh, Jaipur, Rajasthan
Abstract. This paper studies existence and uniqueness of solutions for system of fractional differential equations involving Caputo derivative with anti periodic boundary conditions of order a Î (0.3). We obtain the result by using Banach fixed point theorem.
Keywords. Caputo fractional derivative, fractional differential equations, anti-periodic boundary conditions, Banach fixed point theorem.
1 Introduction
In recent years the subject of fractional calculus gained much momentum and attracted many researchers and mathematicians. Considerable interest in field of fractional calculus has been developed by the applications to different areas of applied science and engineering like physics, biophysics, aerodynamics, control theory, visco-elasticity, capacitor theory, electrical circuit, description of memory and hereditary properties etc.
Anti periodic boundary value problems constitute an important class of boundary value problems and have recently received considerable attention. Anti periodic boundary conditions occur in mathematical modeling of many physical processes, see [6] – [10] and references therein.
The Banach fixed point theorems is used [11] to investigate existence and uniqueness of for integro differential equations of fractional order a Î(1,2) with antiperiodic boundary conditions. In [7] the author investigated existence problem of anti periodic boundary value problem to fractional differential equation for a Î (2,3) by using Banach fixed point. Motivated by these works we study in this paper the existence of solution to fractional differential equation when a Î (0,3] with anti periodic boundary conditions.
Precisely we consider the following problem
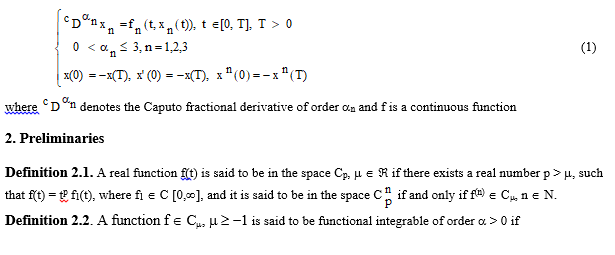






[1] M. Benchohra, S. Hamani, S.K. Ntouyas, Boundary value problems for differential equations with fractional order and nonlocal conditions, Nonlinear Anal., 71 2391-2396 (2000).
[2] K.S. Miller, P.N., B. Ross, An introduction to the fractio;nal calculus and fractional differential equations. Willey, New York (1993)
[3] A.A. Kilbas, H.M. Srivastava, J.J. Trujillo, Theory and applications of fractional differential equations, Elsevier, Amsterdam (2006).
[4] I. Podlubny, Srinivasan, fractional differential equations, Academic Press, New York, (1999).
[5] R. Agarwal, M. Benchohra, S. Hamani, Boundary value problems for fractional differential equations, Georgian mathematicalJournal,16(3): 401-411 (2009).
[6] B. Ahmad, V. Otero Espiner, Existence of solutions for fractional inclusions with anti periodic boundary conditions, Bound. Value Probl.11: Art ID 625347 (2009).
[7] B. Ahmad, Existence of solutions for fractional differential equations of order q Î (2,3] with anti periodic conditions, J. Appl. Math. Comput.,24: 822-825 (2011).
[8] B. Ahmad, J.J. Nieto, Existence of solutions for anti periodic boundary value problems involving fractional differential equations via Larray Shouder degree theory, Topol. Methods Nonlinear Anal.,35: 295-304 (2010).
[9] G. Wang, B. Ahmad, L. Zhang, Impulsive and periodic boundary value problem for nonlinear differential equations of fractional order, Nonlinear Anal.,74: 792-804 (2011).
[10] M. Matar, Existence and uniqueness of solutions to fractional semilinear mixed Volterra Fredholm integrodifferential equations with nonlocal conditions. Electronic Journal of Differential Equations,155: 1-7 ((2009).
[11] D.R.Smart, Fixed Point Theorems, Cambridge University Press (1980).
